Understanding significant figures is crucial for anyone working with measurements and calculations in science, engineering, or mathematics. Significant figures (also called significant digits) communicate the precision of a measurement and ensure that calculations maintain an appropriate level of accuracy. This article explores the concept of significant figures, how to determine the correct number to use, and why they matter in scientific and mathematical contexts.
What Are Significant Figures?
Definition
Significant figures are digits in a number that carry meaningful information about the precision of the measurement.
Significant figures include all digits that are known with certainty plus one digit that contains some uncertainty. They tell us how precise a measurement is and help maintain appropriate precision in calculations.
Example
In the measurement 12.34 cm, all four digits (1, 2, 3, and 4) are significant because they all carry meaningful information about the value.
Rules for Identifying Significant Figures
Rule 1
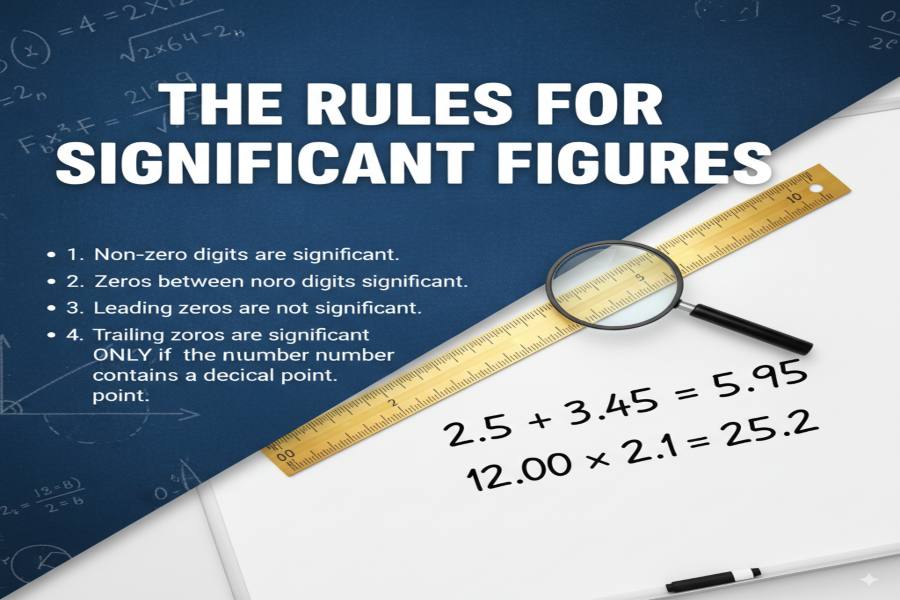
Non-zero digits
All non-zero digits are always significant.
Example: 1234 has 4 significant figures.
Rule 2
Zeros between non-zero digits
Zeros between non-zero digits are always significant.
Example: 1002 has 4 significant figures.
Rule 3
Leading zeros
Zeros to the left of the first non-zero digit are not significant.
Example: 0.00123 has 3 significant figures.
Rule 4
Trailing zeros after decimal
Trailing zeros after the decimal point are significant.
Example: 12.300 has 5 significant figures.
Rule 5
Trailing zeros without decimal
Trailing zeros in a whole number are ambiguous without a decimal point.
Example: 1200 could have 2, 3, or 4 significant figures.
Rule 6
Scientific notation
In scientific notation, all digits shown are significant.
Example: 1.20 × 10³ has 3 significant figures.
Calculations with Significant Figures
Addition and Subtraction
The result should have the same number of decimal places as the measurement with the fewest decimal places.
12.1 + 3.456 = 15.6 (not 15.556)
Multiplication and Division
The result should have the same number of significant figures as the measurement with the fewest significant figures.
4.56 × 1.4 = 6.4 (not 6.384)
Logarithms and Exponents
The number of decimal places in the logarithm equals the number of significant figures in the original number.
log(1.23 × 10⁴) = 4.090
Try it yourself:
Raw result:
-
With correct sig figs:
-
Common Mistakes with Significant Figures
Confusing Precision and Accuracy
Precision refers to how close repeated measurements are to each other, while accuracy refers to how close a measurement is to the true value. Significant figures communicate precision, not accuracy.
Rounding Too Early
Always perform calculations with all available digits and round only the final result to the appropriate number of significant figures.
Misinterpreting Trailing Zeros
Without a decimal point, trailing zeros can be ambiguous. Use scientific notation to clarify the number of significant figures.
Applying Addition/Subtraction Rules to Multiplication/Division
Remember that addition/subtraction uses decimal places, while multiplication/division uses significant figures.
Real-World Applications
Laboratory Science
In chemistry and physics labs, significant figures ensure that experimental results reflect the precision of the measuring instruments used.
Engineering
Engineers use significant figures to ensure that calculations for structures, circuits, and systems maintain appropriate precision.
Pharmaceuticals
Precise measurements are critical in drug formulation, where even small errors can have significant consequences.
Data Analysis
When analyzing experimental data, significant figures help communicate the reliability of results and prevent overstating precision.
"In science, the correct number of significant figures is not just a mathematical nicety—it's an ethical obligation to accurately represent the precision of our knowledge."
Interactive Practice Tool
Test your understanding of significant figures with this interactive tool. Identify the number of significant figures in each value or determine the correct result of calculations.
How many significant figures are in the number: 0.00340?
Conclusion
Understanding the correct number of significant figures is essential for accurate scientific and mathematical work. By following the rules outlined in this article, you can ensure that your calculations maintain an appropriate level of precision and that you neither overstate nor understate the accuracy of your results.
Key Takeaways
- Significant figures communicate the precision of measurements
- Different rules apply for addition/subtraction versus multiplication/division
- Scientific notation can clarify ambiguous cases
- Always perform calculations with all available digits and round only the final result
- Proper use of significant figures is crucial for scientific integrity