SigFig Calculator

Calculate significant figures, convert between notations, and perform operations while preserving sig figs.

Sig Figs: -

Scientific: -

Result: -

sig figs

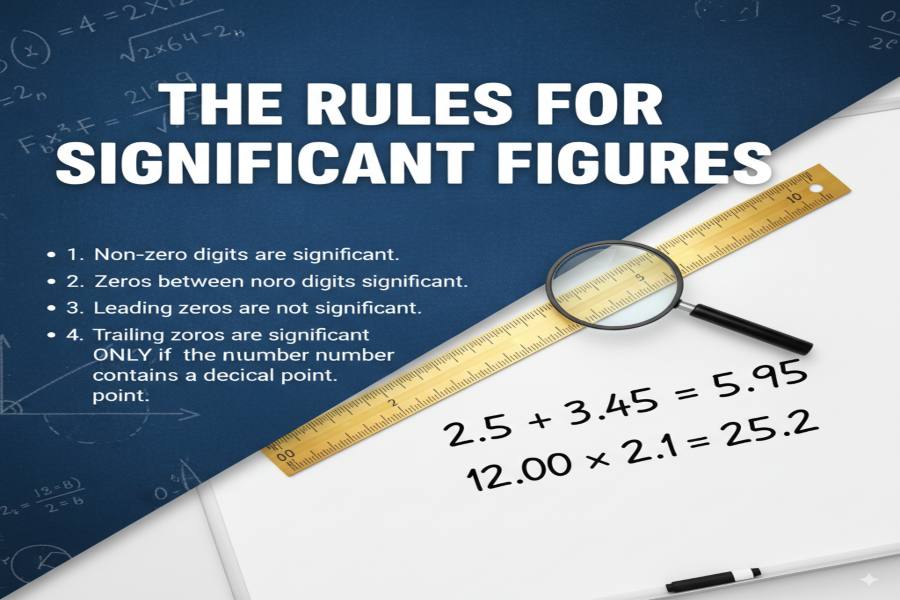
Rule 1: Non-zero digits

All non-zero digits are significant.

Example: 123.45 has 5 significant figures

Rule 2: Zeros between non-zero digits

Zeros between non-zero digits are significant.

Example: 1002 has 4 significant figures

Rule 3: Leading zeros

Leading zeros are never significant.

Example: 0.00123 has 3 significant figures

Rule 4: Trailing zeros

Trailing zeros after a decimal point are significant.

Example: 12.300 has 5 significant figures

Rule 5: Trailing zeros without decimal

Trailing zeros in a whole number are ambiguous unless indicated otherwise.

Example: 1200 has 2-4 significant figures (ambiguous)

Operations with Significant Figures

Addition/Subtraction: Result has the same number of decimal places as the term with the fewest decimal places.
Multiplication/Division: Result has the same number of significant figures as the term with the fewest significant figures.

Key Features

Discover what makes our significant figure calculator the best tool for your scientific calculations

Accurate Counting

Precisely count the number of significant figures in any numerical value, including scientific notation.

Mathematical Operations

Perform addition, subtraction, multiplication, and division while maintaining proper significant figure rules.

Educational Explanations

Learn why a number has a specific count of significant figures with detailed explanations.

Mobile Friendly

Access our calculator on any device with a fully responsive design that works on smartphones, tablets, and desktops.

How To Use Our Calculator

Follow these simple steps to get accurate results with our significant figure calculator

Enter Your Number

Type the number you want to analyze in the input field. You can use standard notation (123.45) or scientific notation (1.2345 × 10^2).

Click Calculate

Press the calculate button to process your input. Our algorithm will analyze the number according to standard significant figure rules.

View Results

The calculator will display the number of significant figures and provide an explanation of how it determined the result.

Try It Now

Significant Figure Examples

Learn through these practical examples how significant figures work in different scenarios

Example 1: Counting Sig Figs

Number: 0.00340

Significant Figures: 3

Leading zeros are not significant. The digits 3, 4, and the trailing 0 are significant because trailing zeros after a decimal point are significant.

Example 2: Scientific Notation

Number: 5.670 × 10^3

Significant Figures: 4

In scientific notation, all digits in the coefficient are significant. Therefore, 5, 6, 7, and 0 are all significant digits.

Example 3: Addition

12.52 + 1.7

Result: 14.2

When adding or subtracting, the result should have the same number of decimal places as the least precise measurement (1.7 has 1 decimal place).

Example 4: Multiplication

2.4 × 3.17

Result: 7.6

When multiplying or dividing, the result should have the same number of significant figures as the measurement with the fewest significant figures (2.4 has 2 sig figs).

Practice Problems

Test your knowledge with these interactive practice problems on significant figures

Problem 1: Counting Significant Figures

How many significant figures are in the number 0.00340?

Problem 2: Addition Rule

What is the result of 23.45 + 1.2 with the correct number of significant figures?

24.65

24.7

24.6

Problem 3: Multiplication Rule

What is the result of 4.2 × 3.156 with the correct number of significant figures?

13.2552

13.26

13.3

Compare Calculators

See how our significant figure calculator compares to other options available online

Features	SigFigCalculator.io	Basic Calculators	Other Sig Fig Tools
Accurate counting of 0.00X numbers
Scientific notation support
Detailed explanations
Mobile responsive design
Addition/subtraction with sig fig rules
Multiplication/division with sig fig rules
Practice problems
Free to use

Scientific Applications

Discover how significant figures are used in various scientific fields

Chemistry

Chemistry Applications

In chemistry, significant figures are crucial for reporting measurements from lab equipment and ensuring accurate calculations in chemical reactions and stoichiometry.

Example: When measuring 25.4 mL of a solution with a graduated cylinder (±0.1 mL precision), the result must be reported with the appropriate significant figures to reflect the measurement uncertainty.

Physics

Physics Applications

Physicists use significant figures to maintain precision in experimental measurements, calculations of physical constants, and theoretical predictions.

Example: When calculating the acceleration due to gravity from experimental data, the number of significant figures in the result must reflect the precision of the measurements used in the calculation.

Engineering

Engineering Applications

Engineers rely on significant figures to ensure precision in design specifications, material properties, and structural calculations.

Example: When designing a bridge, engineers must use appropriate significant figures in stress calculations to ensure safety margins are accurately represented and maintained.

What Our Users Say

Hear from students, teachers, and professionals who use our calculator

This calculator has been a lifesaver for my chemistry students. It not only gives the right answers but explains the reasoning, which helps them learn the concepts better.

Sarah Johnson

Chemistry Teacher

As an engineering student, I use this calculator daily. The interface is intuitive, and it handles scientific notation perfectly. It's become an essential tool for my studies.

Michael Chen

Engineering Student

The best sig fig calculator I've found online. It's accurate, fast, and the explanations help me understand why my answers are right or wrong. Highly recommended!

Emily Rodriguez

Physics Major

Frequently Asked Questions

Find answers to common questions about significant figures and our calculator

What are significant figures?

Significant figures (also called significant digits) are the digits in a number that carry meaningful value and contribute to its precision. They represent the reliability of a measurement or calculation in scientific and engineering applications. The concept helps scientists communicate the precision of their measurements and ensures that calculations don't imply greater accuracy than the original measurements support.

How do I identify significant figures in a number?

Follow these rules to identify significant figures:

All non-zero digits are significant (1-9)
Zeros between non-zero digits are significant
Leading zeros (zeros before the first non-zero digit) are NOT significant
Trailing zeros after a decimal point ARE significant
Trailing zeros in a whole number are ambiguous unless written in scientific notation

Why are significant figures important in science?

Significant figures are crucial in scientific work because they communicate the precision of measurements and prevent false precision in reported results. When scientists perform calculations with measured values, the rules of significant figures ensure that the final result doesn't imply greater accuracy than the original measurements actually had. This maintains scientific integrity and prevents misleading conclusions based on inappropriately precise values.

How do significant figures apply to calculations?

Different operations have different rules:

Addition and Subtraction: The result should have the same number of decimal places as the measurement with the fewest decimal places.
Multiplication and Division: The result should have the same number of significant figures as the measurement with the fewest significant figures.
Logarithms: The number of significant figures in the mantissa (decimal part) of the result equals the number of significant figures in the original number.
Exponentiation: The result should have the same number of significant figures as the base number.