In the world of scientific measurements and calculations, precision matters. Significant figures (also called significant digits) are a crucial concept that helps scientists, engineers, and students communicate the precision of their measurements and calculations. Whether you're working on a chemistry lab report, analyzing physics data, or solving mathematical problems, understanding significant figures is essential for accurate scientific communication.
This comprehensive guide will explore what significant figures are, why they matter, how to determine them, and most importantly, how to use a significant figures calculator to save time and avoid errors. By the end of this article, you'll have a solid understanding of significant figures and be able to apply this knowledge confidently in your scientific work.
What Are Significant Figures?
Significant figures (or sig figs) are the digits in a number that carry meaningful information about the precision of a measurement. They include all digits that are known with certainty plus one digit that contains some uncertainty.
Definition:
Significant figures are all the digits in a measured value that are known with certainty, plus one final digit that is estimated or uncertain.
The Concept of Precision
When we make a measurement, the precision of our measuring instrument determines how many significant figures we can report. For example, if you measure a length with a ruler that has millimeter markings, you can estimate to the nearest 0.1 mm, giving you one more digit of precision than the ruler actually shows.
Consider measuring the length of a pencil:
Example:
If you measure a pencil with a ruler and read 12.7 cm, this value has three significant figures (1, 2, and 7). The digits 1 and 2 are certain based on the ruler's markings, while the 7 represents your best estimate between the millimeter markings.
Why Significant Figures Matter
Understanding and correctly using significant figures is not just a pedantic exercise—it has real implications for scientific work and communication.
Communicating Precision
Significant figures tell others how precise your measurement is. Reporting a value as 10.47 g indicates a much higher precision than reporting it as 10 g.
Avoiding False Precision
Using too many significant figures can imply a level of precision that doesn't actually exist in your measurement, potentially leading to incorrect conclusions.
Propagating Uncertainty
When performing calculations with measured values, the uncertainty in those measurements propagates through the calculation. Significant figures help track this uncertainty.
Scientific Integrity
Properly using significant figures demonstrates scientific integrity by honestly representing the limitations of your data and measurements.
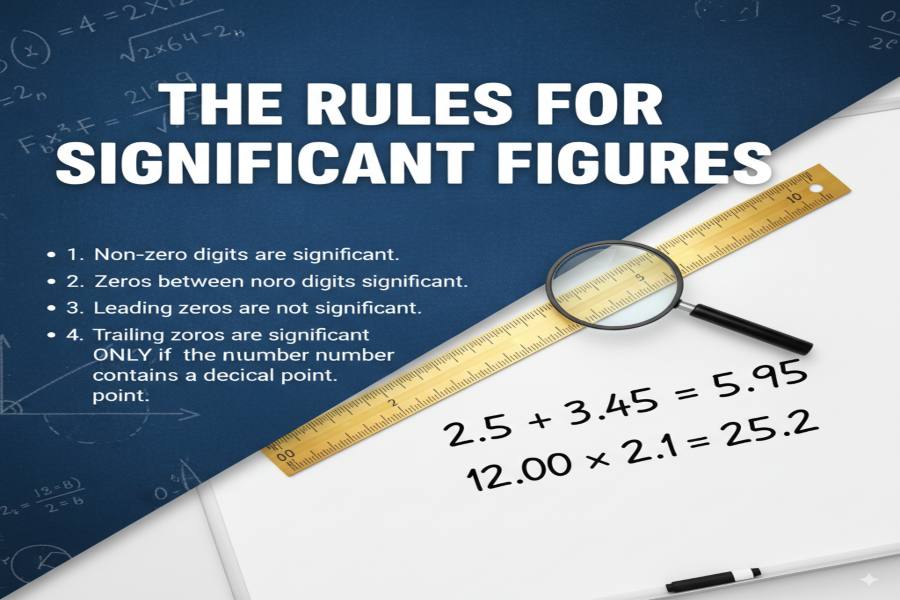
Rules for Determining Significant Figures
To correctly count significant figures, you need to follow these established rules:
1
All Non-Zero Digits Are Significant
Any digit from 1 through 9 is always considered significant.
Example: 1234 has 4 significant figures.
2
Zeros Between Non-Zero Digits Are Significant
Any zero that appears between non-zero digits is significant.
Example: 1002 has 4 significant figures.
3
Leading Zeros Are Never Significant
Zeros that appear before the first non-zero digit are not significant. They merely indicate the position of the decimal point.
Example: 0.00456 has 3 significant figures (4, 5, and 6).
4
Trailing Zeros After a Decimal Point Are Significant
Zeros at the end of a number that contains a decimal point are significant.
Example: 12.300 has 5 significant figures.
5
Trailing Zeros in a Whole Number Are Ambiguous
Without a decimal point, trailing zeros in a whole number may or may not be significant. Scientific notation can be used to clarify.
Example: 1200 could have 2, 3, or 4 significant figures. Writing it as 1.2 × 10³ indicates 2 significant figures.
6
Exact Numbers Have Infinite Significant Figures
Numbers that are defined exactly (like conversion factors or counted objects) have an infinite number of significant figures.
Example: There are exactly 12 inches in 1 foot, so this conversion factor has infinite significant figures.
How to Use a Significant Figures Calculator
A significant figures calculator can save you time and help avoid errors when determining the number of significant figures in a value. Try our calculator below:
Number of Significant Figures:
0
Using a significant figures calculator is straightforward:
- Enter the number you want to analyze in the input field.
- Click the "Calculate Significant Figures" button.
- The calculator will display the number of significant figures and explain how it determined this count.
For more complex calculations involving operations with significant figures, you can use our comprehensive calculator tools that automatically apply the appropriate rounding rules for addition, subtraction, multiplication, and division.
Common Mistakes with Significant Figures
Even experienced scientists and students can make mistakes when working with significant figures. Here are some common pitfalls to avoid:
Confusing Zeros
▼
One of the most common mistakes is misinterpreting the significance of zeros. Remember that leading zeros are never significant, zeros between non-zero digits are always significant, and trailing zeros after a decimal point are significant.
Example: In 0.0120, there are 3 significant figures (1, 2, and the trailing 0), not 5.
Forgetting to Round Properly
▼
After performing calculations, many people forget to round their final answer to the appropriate number of significant figures based on the input values.
Example: If you multiply 2.3 cm (2 sig figs) by 4.56 cm (3 sig figs), your calculator might show 10.488 cm², but the correct answer with proper significant figures is 10 cm² (2 sig figs).
Applying the Wrong Rule for Different Operations
▼
Addition/subtraction and multiplication/division have different rules for determining significant figures in the result.
For addition/subtraction: The result should have the same number of decimal places as the measurement with the fewest decimal places.
For multiplication/division: The result should have the same number of significant figures as the measurement with the fewest significant figures.
Ambiguity with Trailing Zeros in Whole Numbers
▼
Without a decimal point, it's unclear whether trailing zeros in a whole number are significant. For example, 1200 could have 2, 3, or 4 significant figures.
Solution: Use scientific notation to clarify. 1.2 × 10³ has 2 significant figures, 1.20 × 10³ has 3, and 1.200 × 10³ has 4.
Treating Exact Numbers Incorrectly
▼
Exact numbers (like conversion factors or counted objects) have infinite significant figures and don't limit the precision of your result.
Example: When converting 2.45 meters to centimeters using the exact conversion factor (1 m = 100 cm), the result is 245 cm with 3 significant figures, not 2 significant figures.
Examples of Significant Figures in Calculations
Let's explore some practical examples of how significant figures are applied in various calculations:
Addition & Subtraction
Multiplication & Division
Mixed Operations
Scientific Notation
Example 1: Adding measurements with different precisions
Calculate the sum of 12.3 cm + 5.67 cm + 8 cm
12.3 cm (1 decimal place)
5.67 cm (2 decimal places)
8 cm (0 decimal places)
-------------------------
Sum = 25.97 cm
Since the least precise measurement (8 cm) has 0 decimal places, the final answer should have 0 decimal places:
Final answer: 26 cm
5.67 cm (2 decimal places)
8 cm (0 decimal places)
-------------------------
Sum = 25.97 cm
Since the least precise measurement (8 cm) has 0 decimal places, the final answer should have 0 decimal places:
Final answer: 26 cm
Example 2: Multiplying measurements with different significant figures
Calculate the area of a rectangle with length 4.56 m and width 2.1 m
Length = 4.56 m (3 significant figures)
Width = 2.1 m (2 significant figures)
Area = Length × Width
Area = 4.56 m × 2.1 m = 9.576 m²
Since the measurement with the fewest significant figures (width) has 2 significant figures, the final answer should have 2 significant figures:
Final answer: 9.6 m²
Width = 2.1 m (2 significant figures)
Area = Length × Width
Area = 4.56 m × 2.1 m = 9.576 m²
Since the measurement with the fewest significant figures (width) has 2 significant figures, the final answer should have 2 significant figures:
Final answer: 9.6 m²
Example 3: Combining addition and multiplication
Calculate (3.14 × 2.5) + 7.1
First, perform the multiplication:
3.14 (3 significant figures) × 2.5 (2 significant figures) = 7.85
This result should have 2 significant figures: 7.9
Next, perform the addition:
7.9 (1 decimal place) + 7.1 (1 decimal place) = 15.0
Since both numbers in the addition have 1 decimal place, the final answer should have 1 decimal place:
Final answer: 15.0
3.14 (3 significant figures) × 2.5 (2 significant figures) = 7.85
This result should have 2 significant figures: 7.9
Next, perform the addition:
7.9 (1 decimal place) + 7.1 (1 decimal place) = 15.0
Since both numbers in the addition have 1 decimal place, the final answer should have 1 decimal place:
Final answer: 15.0
Example 4: Working with scientific notation
Calculate (2.5 × 10⁻³) × (4.0 × 10⁵)
2.5 × 10⁻³ (2 significant figures)
4.0 × 10⁵ (2 significant figures)
(2.5 × 10⁻³) × (4.0 × 10⁵) = (2.5 × 4.0) × (10⁻³ × 10⁵) = 10.0 × 10² = 1.00 × 10³
Since both input values have 2 significant figures, the final answer should have 2 significant figures:
Final answer: 1.0 × 10³
4.0 × 10⁵ (2 significant figures)
(2.5 × 10⁻³) × (4.0 × 10⁵) = (2.5 × 4.0) × (10⁻³ × 10⁵) = 10.0 × 10² = 1.00 × 10³
Since both input values have 2 significant figures, the final answer should have 2 significant figures:
Final answer: 1.0 × 10³
Advanced Applications of Significant Figures
Beyond basic calculations, significant figures play a crucial role in various scientific and engineering applications:
Laboratory Analysis
In analytical chemistry, significant figures are essential when reporting concentrations, masses, and volumes. The precision of instruments like balances and pipettes determines how many significant figures can be reported.
Research Publications
Scientific journals often have specific requirements for reporting numerical data with appropriate significant figures to ensure the integrity and reproducibility of research findings.
Manufacturing Tolerances
In engineering and manufacturing, significant figures help communicate the precision required for parts and components, ensuring they meet design specifications and quality standards.
Data Analysis
When analyzing experimental data, proper use of significant figures helps prevent overinterpretation of results and ensures that conclusions are supported by the actual precision of the measurements.
Error Propagation
Advanced scientific work often requires formal error propagation calculations. Significant figures provide a simplified approach to tracking uncertainty through calculations.
Education
Teaching significant figures helps students develop critical thinking about measurement precision and uncertainty, fundamental concepts in scientific literacy.
Test Your Knowledge
Put your understanding of significant figures to the test with these practice problems:
How many significant figures are in the number 0.00340?
Conclusion
Understanding significant figures is a fundamental skill for anyone working in science, engineering, or mathematics. By properly applying the rules of significant figures, you can communicate the precision of your measurements and calculations accurately, avoiding both false precision and unnecessary vagueness.
A significant figures calculator is a valuable tool that can help you quickly determine the number of significant figures in a value and ensure that your calculations follow the proper rules for significant figures. By using such tools and understanding the underlying principles, you can enhance the quality and integrity of your scientific work.
Remember that significant figures are more than just a mathematical convention—they represent our honest assessment of how precisely we know a value. This honesty is at the heart of good scientific practice and is essential for building reliable knowledge.